捕食-食饵模型是种群动力学的重要研究内容,吸引了国内外众多学者的关注.最早由Volterra在1926年提出了Lotka-Volterra模型[1],但此模型假设功能反应与食饵数量成正比,这与实际情况不完全相符.随后Holling在实验的基础上对不同物种提出了3种不同的功能反应函数.在食饵与捕食者的相互作用中,很多食饵是具有防卫能力的,特别是随着食饵数量的增加,食饵的防御、匿藏能力也会提高,对捕食者会起到抑制作用.基于此,Andrews提出了Holling Ⅳ型[2]功能反应函数P(x)=mx/a+bx+x2.在现实生产生活中,考虑到经济利益,往往会对食饵进行收获以出售,因此对捕食-食饵模型考虑进收获项是很有必要的,而Michaelis-Menten收获项从生态和经济的角度都更加符合实际[3, 4, 5].
目前已有许多学者对带收获项的捕食-食饵模型进行了研究,并取得大量成果.其中,文献[6]研究了带常数收获项的Holling-Ⅳ型捕食-食饵模型,证明了模型的多种分歧情况;文献[7]中提出了带有Michaelis-Menten收获项的改进的HollingⅡ型捕食-食饵模型,并研究了解的稳定性和分歧;文献[8]通过对文献[7]中的模型添加扩散项和扩散系数,研究了解的渐近稳定性,周期解的性态和非常数正解的存在性等. 结合文献[6, 7, 8],本文考虑如下一类在齐次Neumann边界条件下带Michaelis-Menten收获项的改进的Holling-Ⅳ型捕食-食饵模型
| \[\left\{ {\begin{array}{*{20}{l}} {{u_t} - {d_1}\Delta u = u(1 - u) - \frac{{au\upsilon }}{{m + u + {u^2}}} - \frac{{hu}}{{c + u}},}&{x \in \Omega ,t > 0,}\\ {{\upsilon _t} - {d_2}\Delta \upsilon = \rho \upsilon \left( {1 - \frac{{\beta \upsilon }}{{m + u + {u^2}}}} \right),}&{x \in \Omega ,t > 0,}\\ {{\partial _\upsilon }u = {\partial _\upsilon }\upsilon = 0,}&{x \in \partial \Omega ,t > 0,}\\ {u(x,0) = {u_0} \ge 0,\upsilon (x,0) = {\upsilon _0},}&{x \in \Omega .} \end{array}} \right.\] | (1) |
其中u,v分别代表食饵和捕食者的种群密度,Δ为Lapalce算子,$\partial $v表示单位外法向量的方向导数,Ω为RN(N≥1)中具有光滑边界的有界开区域,d1>0和d2>0分别是食饵和捕食者的扩散系数,α,β,γ,ρ,h,m,c均为正常数.
本文主要借助L-S度理论等,研究系统(1)的共存解,因此考虑对应的平衡态方程
| \[\left\{ {\begin{array}{*{20}{l}} { - {d_1}\Delta u = u(1 - u) - \frac{{au\upsilon }}{{m + u + {u^2}}} - \frac{{hu}}{{c + u}},}&{x \in \Omega ,}\\ { - {d_2}\Delta \upsilon = \rho \upsilon \left( {1 - \frac{{\beta \upsilon }}{{m + u + {u^2}}}} \right),}&{x \in \Omega ,}\\ {{\partial _\upsilon }u = {\partial _\upsilon }\upsilon = 0,}&{x \in \partial \Omega .} \end{array}} \right.\] | (2) |
令0=λ0<λ1<λ2<…→∞是-Δ算子在Ω上关于齐次Neumann边界条件下的特征值,$\left\{ {{\phi _{ij}}} \right\}\mathop {\dim \left\langle {({\lambda _i})} \right\rangle }\limits_{j = 1} $是E(λi)={ψ|-Δφ=λiφ,x∈Ω,$\partial $vφ=0,x∈$\partial $Ω}的一组标准正交基.设X={(u,v)∈[C1(Ω)]2|$\partial $vu=$\partial $vv=0,x∈$\partial $Ω},Xij={cφij|c∈R2},则
| \[X = \mathop \oplus \limits_{i = 1}^ \propto {X_i},{X_i} = \mathop \oplus \limits_{j = 1}^{\dim \left\{ {\left( {{\lambda _i}} \right)} \right\}} {X_{ij}}.\] |
对于系统(2)非负常数解的情况有如下定理.
定理1 对于系统(2),令s=1-c-α/β,Δ0=(α/β-c-1)2-4h,则有
(1) (0,0)为其平凡解,(0,m/β)为其一个非负半平凡解.
(2) 当h>c,c<1且(1+c)2>4h时,有两个半平凡解
| \[{U_A} = \left( {{u_A},0} \right) = \left( {\frac{{1 - c - \sqrt {{{\left( {1 - c} \right)}^2} - 4h} }}{2},0} \right),{U_B} = \left( {{u_B},0} \right) = \left( {\frac{{1 - c - \sqrt {{{\left( {1 - c} \right)}^2} - 4h} }}{2},0} \right);\] |
当h<c时,仅有UB=(uB,0)存在.
(3) 当1-h/c<α/β<1+c-2$\sqrt h $<1-c时,有两个正常数解
| \[{U_1} = \left( {{u_1},{\upsilon _1}} \right) = \left( {\frac{{s - \sqrt {{\Delta _0}} }}{2},\frac{{m + {u_1} + u_1^2}}{\beta }} \right),{U_2} = \left( {{u_2},{\upsilon _2}} \right) = \left( {\frac{{s + \sqrt {{\Delta _0}} }}{2},\frac{{m + {u_2} + u_2^2}}{\beta }} \right);\] |
当α/β<1-h/c时,仅有U2=(u2,v2)存在.
(4) 当1-h/c<α/β=1+c-2$\sqrt h $<1-c时,只有一个正常数解$\bar U = \left( {\bar u,\bar \upsilon } \right) = \left( {\frac{s}{2},\frac{{m + \bar u + {{\bar u}^2}}}{\beta }} \right)$.
(5) 当α/β>1+c-2$\sqrt h $时,无正常数解.
由最大值原理[9]可以得到如下定理.
定理2 假设(u,v)是系统(2)的任意非负解,且u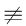 0,v
0,v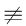 0,则$u \le 1,\frac{m}{\beta } \le \upsilon \le \frac{{m + r + 1}}{\beta }.$
0,则$u \le 1,\frac{m}{\beta } \le \upsilon \le \frac{{m + r + 1}}{\beta }.$
证明 令
| \[u({x_1}) = \mathop {\max }\limits_{\bar \Omega } u,\upsilon ({y_2}{\rm{) = }}\mathop {\max }\limits_{\bar \Omega } \upsilon .\] |
对于系统(1),由最大值原理可得
| \[\begin{array}{l} 0 \le u({x_1})\left[ {1 - u({x_1})} \right] - \frac{{a\upsilon ({x_1})\upsilon ({x_1})}}{{m + u({x_1}) + {u^2}({x_1})}} - \frac{{hu({x_1})}}{{c + u({x_1})}} \le u({x_1})\left[ {1 - u({x_1})} \right],\\ \rho \upsilon ({y_2}{\rm{)}}\left[ {1 - \frac{{\beta \upsilon ({y_2}{\rm{)}}}}{m}} \right] \le \rho \upsilon ({y_2}{\rm{)}}\left[ {1 - \frac{{\beta \upsilon ({y_2}{\rm{)}}}}{{m + ru({y_2}{\rm{)}} + {u^2}({y_2}{\rm{)}}}}} \right] \le 0, \end{array}\] |
因此有u(x1)≤1,v(y2)≥m/β.
同理,令v(x2)=maxΩ v,则有
| \[\begin{array}{l} \\ 0 \le \rho \upsilon ({x_2}{\rm{)}}\left[ {1 - \frac{{\beta \upsilon ({x_2}{\rm{)}}}}{{m + ru({x_2}{\rm{)}} + {u^2}({x_2}{\rm{)}}}}} \right] \le \rho \upsilon ({x_2}{\rm{)}}\left[ {1 - \frac{{\beta \upsilon ({x_2}{\rm{)}}}}{{m + r + 1}}} \right], \end{array}\] |
所以$\upsilon ({x_2}{\rm{)}} \le \frac{{m + r + 1}}{\beta }.$
由Harnack不等式[10]可得如下定理.记Λ表示参数α,β,γ,c,h,m,ρ的集合,下文所涉及的常数C,C*,C都与区域Ω和参数Λ有关.
定理3 设α/β≠1-h/c,d是固定的正常数,则存在一个正常数C=C(Λ,d),使得对所有的d1,d2≥d,系统(2)的任意正解(u,v)满足u(x)≥C,v(x)≥m/β.
证明 令${c_1}(x) = \frac{1}{{{d_1}}}\left( {1 - u - \frac{{\alpha \upsilon }}{{m + u + {u^2}}} - \frac{h}{{c + u}}} \right),{c_2}(x) = \frac{1}{{{d_2}}}\left( {\rho - \frac{{\beta \alpha \upsilon }}{{m + u + {u^2}}}} \right).$
结合定理2可知,存在一个正常数C=C(N,Ω,Λ,d),使得当d1,d2≥d时,有‖c1(x)‖∞,‖c2(x)‖∞≤C.因此,若u满足
| \[\left\{ {\begin{array}{*{20}{l}} {\Delta u + c(x)u = 0,}&{x \in \Omega ,}\\ {{\partial _\upsilon }u = 0,}&{x \in \partial \Omega ,} \end{array}} \right.\] |
则由Harnac不等式可知,存在一个正常数C*=C*(N,Ω,Λ,d),使得当d1,d2≥d时,有
| \[\mathop {\max }\limits_{\bar \Omega } u \le {C^ * }\mathop {\min }\limits_{\bar \Omega } u.\] | (3) |
假设u(x)≥C不成立,则由式(3)可知,存在一个序列{(d1j,d2j)}∞j=1,满足d1j≥d,d2j≥d,且当di=dij,i=1,2时,系统(2)对应的正解(uj,vj)满足当j→∞时,$\mathop {\min }\limits_{\bar \Omega } {u_j} \to 0$.
令${\omega _j} = \frac{{{u_j}}}{{||{u_j}|{|_{{L^ \propto }}}}}$,显然(wj,uj,vj)满足
| \[\left\{ {\begin{array}{*{20}{l}} {\int {_\Omega {\omega _j}\left( {1 - {u_j} - \frac{{\alpha {\upsilon _j}}}{{m + r{u_j} + u_j^2}} - \frac{h}{{c + {u_j}}}} \right){\rm{d}}x = 0,} }\\ {\int {_\Omega \rho {\upsilon _j}\left( {1 - \frac{{\beta {\upsilon _j}}}{{m + r{u_j} + u_j^2}}} \right){\rm{d}}x = 0.} } \end{array}} \right.\] | (4) |
根据Sobolev嵌入定理和椭圆方程的正则性估计[11]可知,存在{uj,vj}j=1∞的一个收敛子序列,为便于研究,仍记为{uj,vj}j=1∞,则当j→∞时,存在两个非负的函数$\tilde u,\tilde \upsilon \in {C^2}(\Omega )$和d0,使得
| \[\left( {{u_j},{\upsilon _j}} \right) \to \left( {\tilde u,\tilde \upsilon } \right) \in {C^2}(\Omega ) \times {C^2}(\Omega ),\left( {{d_0},{d_0}} \right) \in [d,\infty ) \times [d,\infty ).\] |
容易得到$\left( {\tilde u,\tilde \upsilon } \right)$也满足定理2中的估计,且$\mathop {\min }\limits_{\bar \Omega } \tilde u = 0$,故由Harnac不等式可得$\tilde u \equiv 0$.又‖w‖L∞=1,$\tilde \upsilon = m/\beta $,所以结合式(4)的第一式,当j→∞时,有α/β=1-h/c,这与假设条件矛盾,因此u(x)≥C.
2 非常数正平衡解的不存在性定理4 存在一个正常数D1,使得当d1,d2≥D1时,系统(1)没有非常数正平衡解.
证明 假设系统(1)存在非常数正平衡解(u,v),令$\bar u = \frac{1}{{|\Omega |}}\int {_\Omega u} {\rm{d}}x,\tilde \upsilon = \frac{1}{{|\Omega |}}\int {_\Omega u} {\rm{d}}x$,则有
| \[\int {_\Omega (u - \bar u)} {\rm{d}}x = \int {_\Omega (\upsilon - \bar \upsilon )} {\rm{d}}x = 0.\] |
对系统(2)的第一个方程乘以$\left( {u - \bar u} \right)$,第二个方程乘以$\left( {\upsilon - \bar \upsilon } \right)$,并分别在Ω上积分后再相加可得
| \[\begin{array}{*{20}{l}} {\int {_\Omega ({d_1}|\nabla u|{|^2} + {d_2}|\nabla u{|^2})} {\rm{d}}x = }&{ = \int {_\Omega \left\{ {(u - \bar u)\left. {\left[ {u - (1 - u) - \frac{{\alpha u\upsilon }}{{m + ru + {u^2}}} - \frac{{hu}}{{c + u}}} \right]} \right\}{\rm{d}}x + } \right.} }\\ {}&{\int {_\Omega \left\{ {(\upsilon - \bar \upsilon )\left. {\left[ {\rho \upsilon (1 - \frac{{\beta \upsilon }}{{m + ru + {u^2}}})} \right]} \right\}{\rm{d}}x = } \right.} }\\ {}&{\int {_\Omega \left[ {u(u - \bar u)(1 - u - \frac{h}{{c + u}}) - (u - \bar u)\left( {\frac{{\alpha u}}{{m + ru + {u^2}}}} \right)\upsilon + } \right.} }\\ {}&{\left. {\upsilon (\upsilon - \bar \upsilon )\left( {\rho - \frac{{\beta \rho \upsilon }}{{m + ru + {u^2}}}} \right)} \right]{\rm{d}}x.} \end{array}\] |
令${P_1}(u) = \left( {1 - u - \frac{h}{{c + u}}} \right),{P_2}(u) = \frac{{\alpha u}}{{m + ru + {u^2}}},{P_3}(u) = \frac{{\beta \rho \upsilon }}{{m + ru + {u^2}}},$则
| \[\begin{array}{*{35}{l}} \int{_{\Omega }({{d}_{1}}|\nabla u{{|}^{2}}+{{d}_{2}}|\nabla u{{|}^{2}})}\text{d}x= \\ \int{_{\Omega }\left[ u(u-\bar{u}){{P}_{1}}(u)-(u-\bar{u}){{P}_{2}}(u)\upsilon +\upsilon (\upsilon -\bar{\upsilon })(\rho -{{P}_{3}}(u,\upsilon )) \right]\text{d}x=} \\ \int{_{\Omega }\left[ (u-\bar{u})(u{{P}_{1}}(u)-\upsilon {{P}_{2}}(u)-\bar{u}{{P}_{1}}(u)+\bar{\upsilon }{{P}_{2}}(u))+ \right.} \\ \left. (\upsilon -\bar{\upsilon })(\rho \upsilon -\upsilon {{P}_{3}}(u,\upsilon )-\rho \bar{\upsilon }+\bar{\upsilon }{{P}_{3}}(\bar{u},\bar{\upsilon }) \right]\text{d}x= \\ \int{_{\Omega }\left\{ (u-\bar{u})\left[ {{P}_{1}}(u)(u-\bar{u})+\bar{u}{{P}_{1}}(u)-{{P}_{1}}(\bar{u})-{{P}_{2}}(u)(\upsilon -\bar{\upsilon })-\bar{\upsilon }{{P}_{2}}(u){{-}_{2}}(u)) \right] \right\}\text{d}x+} \\ \int{_{\Omega }\left[ \rho {{(\upsilon -\bar{\upsilon })}^{2}}-(\upsilon -\bar{\upsilon })(\upsilon {{P}_{3}}(u,\upsilon )-\bar{\upsilon }{{P}_{3}}(\bar{u},\bar{\upsilon })) \right]\text{d}x=} \\ \int{_{\Omega }\left\{ (u-\bar{u})\left[ {{P}_{1}}(u)(u-\bar{u})+\bar{u}(u-\bar{u}){{P}_{1}}u(\xi )-{{P}_{2}}(u)(\upsilon -\bar{\upsilon })-\bar{\upsilon }(u-\bar{u}){{P}_{2}}u(\eta ) \right] \right\}\text{d}x+} \\ \int{_{\Omega }\left\{ \rho {{(\upsilon -\bar{\upsilon })}^{2}}-(\upsilon -\bar{\upsilon })\left[ {{P}_{3}}(\bar{u},\bar{\upsilon })(\upsilon -\bar{\upsilon })+\bar{\upsilon }{{P}_{3}}(\bar{u},\bar{\upsilon })-{{P}_{3}}(\bar{u},\bar{\upsilon })) \right] \right\}\text{d}x=} \\ \int{_{\Omega }\left\{ (u-\bar{u})\left[ {{P}_{1}}(u)(u-\bar{u})+\bar{u}(u-\bar{u}){{P}_{1}}u(\xi )-{{P}_{2}}(u)(\upsilon -\bar{\upsilon })-\bar{\upsilon }(u-\bar{u}){{P}_{2}}u(\eta ) \right] \right\}\text{d}x+} \\ \int{_{\Omega }\left\{ \rho {{(\upsilon -\bar{\upsilon })}^{2}}-(\upsilon -\bar{\upsilon })\left[ {{P}_{3}}(u,\upsilon )(\upsilon -\bar{\upsilon })+\bar{\upsilon }((u-\bar{u}){{P}_{3}}u({{u}^{\prime }},{{\upsilon }^{\prime }})) \right] \right\}\text{d}x=} \\ \int{_{\Omega }\left[ {{(u-\bar{u})}^{2}}{{P}_{1}}(u)+\bar{u}{{P}_{1}}u(\xi )-\bar{\upsilon }{{P}_{2}}u(\eta )+{{(\upsilon -\bar{\upsilon })}^{2}}\rho -{{P}_{3}}(u,\upsilon )-\bar{\upsilon }{{P}_{3}}u({{u}^{\prime }},{{\upsilon }^{\prime }})) \right]}\text{d}x+ \\ \int{_{\Omega }\left[ (u-\bar{u})(\upsilon -\bar{\upsilon })(-{{P}_{2}}(u)-\bar{\upsilon }{{P}_{3}}u({{u}^{\prime }},{{\upsilon }^{\prime }}) \right]\text{d}x\le } \\ \int{_{\Omega }\left[ {{(u-\bar{u})}^{2}}({{P}_{1}}({{u}^{\prime\prime }})+\bar{u}{{M}_{1}}+\bar{\upsilon }{{M}_{2}}+{{(\upsilon -\bar{\upsilon })}^{2}}\rho +|u-\bar{u}||\upsilon -\bar{\upsilon }|({{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}}) \right]\text{d}x\le } \\ \int{_{\Omega }\left\{ {{(u-\bar{u})}^{2}}\left( {{P}_{1}}({{u}^{\prime\prime }})+\bar{u}{{M}_{1}}+\bar{\upsilon }{{M}_{2}}+\frac{{{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}}}{2\xi } \right)+{{(\upsilon -\bar{\upsilon })}^{2}}\left[ \rho +\frac{\xi ({{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}})}{2} \right] \right\}\text{d}x.} \\ \end{array}\] | (5) |
其中min{C,m/β}≤ξ,η,u′,v′≤max{1,(m+γ+1)/β},M1=|P1u(ξ)|,M2=|P2u(η)|,M3=|P3u(u′,v′)|为固定正常数,ζ为足够小的正常数,u″=$\sqrt{h}$-c为P1(u)的最大值点.所以由Poincare不等式并结合式(5)可得
| \[\begin{align} & \int{_{\Omega }}\left[ {{d}_{1}}{{\lambda }_{1}}{{(u-\bar{u})}^{2}}+{{d}_{2}}{{\lambda }_{2}}{{(\upsilon -\bar{\upsilon })}^{2}} \right]\text{d}x\le \\ & \int{_{\Omega }}\left\{ {{(u-\bar{u})}^{2}}\left( {{P}_{1}}({{u}^{\prime\prime }})+\bar{u}{{M}_{1}}+\bar{\upsilon }{{M}_{2}}+\frac{{{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}}}{2\xi } \right)+{{(\upsilon -\bar{\upsilon })}^{2}}\left[ \rho +\frac{\xi ({{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}})}{2} \right] \right\}\text{d}x. \\ \end{align}\] |
令
| \[\begin{align} & {{D}_{1}}=\max \left\{ \frac{1}{{{\lambda }_{1}}}\left[ {{P}_{1}}({{u}^{\prime\prime }})+\bar{u}{{M}_{1}}+\bar{\upsilon }{{M}_{2}}+\frac{{{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}}}{2\xi } \right] \right\}, \\ & \frac{1}{{{\lambda }_{1}}}\left[ \rho +\frac{\xi {{P}_{2}}(\sqrt{m})+\bar{\upsilon }{{M}_{3}}}{2} \right], \\ \end{align}\] |
所以存在正常数D1>0,使得当d1,d2≥D1时,有$u\equiv \bar{u},\upsilon \equiv \bar{\upsilon }$,矛盾,故定理4得证.
3 非常数正平衡解的全局存在性本节固定其他参数,以d1,d2为参数讨论系统(1)的非常数正平衡解的全局存在性.
首先,记U=(u,v),Ur=(ur,vr),且r=1,2.令
| \[\begin{align} & D=\left( \begin{array}{*{35}{l}} {{f}_{u}} & {{f}_{\upsilon }} \\ {{g}_{u}} & {{g}_{\upsilon }} \\ \end{array} \right)\left| _{({{u}_{r}},{{\upsilon }_{r}}}\text{=} \right.\left( \begin{array}{*{35}{l}} {{f}_{r1}} & {{f}_{r2}} \\ {{g}_{r1}} & {{g}_{r2}} \\ \end{array} \right)= \\ & \left( \begin{matrix} {{u}_{r}}\left( -1+\frac{1}{\beta (m+r{{u}_{r}}+u_{r}^{2})}+\frac{h}{{{(c+{{u}_{r}})}^{2}}} \right) & \frac{-\alpha {{u}_{r}}}{m+r{{u}_{r}}+u_{r}^{2}} \\ \frac{\rho (2{{u}_{r}}+r)}{\beta } & -\rho \\ \end{matrix} \right). \\ \end{align}\] |
经计算可得
| \[\begin{array}{l} {f_{11}}{g_{12}} - {f_{12}}{g_{11}} = - \frac{{\rho {u_1}}}{{c + {u_1}}}\sqrt {{{\left( {\frac{\alpha }{\beta } - c - 1} \right)}^2} - 4h} < 0,\\ {f_{21}}{g_{22}} - {f_{22}}{g_{21}} = - \frac{{\rho {u_2}}}{{c + {u_2}}}\sqrt {{{\left( {\frac{\alpha }{\beta } - c - 1} \right)}^2} - 4h} > 0. \end{array}\] |
则系统(2)可以写成
| \[\left\{ \begin{align} & -\Delta U={{D}^{-1}}F\left( U \right),x\in \Omega , \\ & {{\partial }_{v}}U=0,x\in \partial \Omega . \\ \end{align} \right.\] | (6) |
并且U是系统(2)的解当且仅当U满足
G(d1,d2;U)=U-(I-Δ)-1(D-1F(U)+U)=0,U∈X.
其中(I-Δ)-1是I-Δ在齐次Neumann边界条件下的逆算子.通过计算得
| \[{{D}_{U}}G({{d}_{1}},{{d}_{2}};{{U}_{r}})=I-{{(I-\Delta )}^{-1}}({{D}^{-1}}{{J}_{r}}\left( U \right)+I),r=1,2.\] | (7) |
易知,对于每个Xi,μ是dUG(d1,d2;Ur)在Xi上的特征值,当且仅当μ(1+λi)是矩阵
| \[{{R}_{r}}({{\lambda }_{i}})={{\lambda }_{i}}I-{{D}^{-1}}{{J}_{r}}=\left\{ \begin{matrix} {{\lambda }_{i}}-{{d}^{-1}}_{1}{{f}_{r1}} & -{{d}^{-1}}_{1}{{f}_{r2}} \\ -{{d}^{-1}}_{2}{{g}_{r1}} & {{\lambda }_{i}}-{{d}^{-1}}_{2}{{g}_{r2}} \\ \end{matrix} \right\}\] |
Mr(d1,d2;λi)=d1d2detRr(λi),r=1,2,
所以有
Mr(d1,d2;λ)=d1d2(λ-fr1d1-1)(λ-gr2d2-1)-fr2gr1=
d1d2λ2-(d2fr1+d1gr2)λ+fr1gr2-fr2gr1.
(8)
关于λ的一元二次方程(8)的判别式记为Δr,其中
Δr=(d2fr1+d1gr2)2-4d1d2(fr1gr2-fr2gr1)=
fr12d22+2(2fr2gr1-fr1gr2)d1d2+d12gr22.
则必存在${{{\tilde{d}}}_{2}}$>0,使得当d2>${{{\tilde{d}}}_{2}}$时,Δr大于0,此时Mr有两个根,记为
| \[\begin{align} & {{\lambda }^{+}}_{r}({{d}_{1}},{{d}_{2}})=\frac{1}{2{{d}_{1}}{{d}_{2}}}({{d}_{2}}{{f}_{r1}}+{{d}_{1}}{{g}_{r2}}+\sqrt{{{({{d}_{2}}{{f}_{r1}}+{{d}_{1}}{{g}_{r2}})}^{2}}-4{{d}_{1}}{{d}_{2}}({{f}_{r1}}{{g}_{r}}2-{{f}_{r2}}{{g}_{r1}})),} \\ & {{\lambda }^{+}}_{r}({{d}_{1}},{{d}_{2}})=\frac{1}{2{{d}_{1}}{{d}_{2}}}({{d}_{2}}{{f}_{r1}}+{{d}_{1}}{{g}_{r2}}-\sqrt{{{({{d}_{2}}{{f}_{r1}}+{{d}_{1}}{{g}_{r2}})}^{2}}-4{{d}_{1}}{{d}_{2}}({{f}_{r1}}{{g}_{r2}}-{{f}_{r2}}{{g}_{r1}})).} \\ \end{align}\] |
令
Ar=Ar(d1,d2)={λ|λ≥0,λ+r(d1,d2)<λ<λ+r(d1,d2)},Sp={λ0,λ1,λ2,…}.
引理 1[15] 假设∀λi∈Sp,Mr(d1,d2;λi)≠0且r=1,2,则
index(G(d1,d2;·),Ur)=(-1)σ,
其中
| \[\sigma =\left\{ \begin{align} & \sum\limits_{{{\lambda }_{i}}\in {{A}_{r}}\cap {{S}_{p}}}{dimE({{\lambda }_{i}}),{{A}_{r}}\cap {{S}_{p}}\ne \varnothing } \\ & 0,{{A}_{r}}\cap {{S}_{p}}=\varnothing \\ \end{align} \right.\] |
特别地,若∀λi≥0,有Mr(d1,d2;λi)>0,则σ=0.
定理 5 设1-h/c<α/β<1+c-2$\sqrt{h}$<1-c成立,且f21<0.如果d1固定,使得∀q≥1都有$\frac{{{f}_{11}}}{{{d}_{1}}}\in ({{\lambda }_{q}},{{\lambda }_{q}}+1),{{\sigma }_{q}}=\sum\limits_{i=1}^{q}{dimE({{\lambda }_{i}})}$为奇数,则存在一个正常数d2*,使得当d2≥d2*时,系统(1)至少存在一个非常数正平衡解.
证明 因为f11g12-f12g11<0,所以λ1+>0,λ1-<0.又$\underset{{{d}_{2}}\to \infty }{\mathop{\lim }}\,{{\lambda }^{+}}_{1}=\frac{{{f}_{11}}}{{{d}_{1}}}>0$,从而存在${{{\hat{d}}}_{2}}$>0,使得当d2≥${{{\hat{d}}}_{2}}$时,有λq<λ1+<λq+1.故当i≤q时,M1(d1,d2;λi)<0;当i≥q+1时,M1(d1,d2;λi)>0.所以A1(d1,d2)∩Sp={λ0,λ1,λ2,…λq}.
又因为f21<0,g22<0,f21g22-f22g21>0,可以得到λ2+<0,λ2-<0,从而∀λi≥0,有M2(d1,d2;λ)>0,故σ=0.
接下来令
| \[{{d}^{*}}_{2}=max\left\{ {{{\hat{d}}}_{2}},{{{\hat{d}}}_{2}},\frac{1}{{{\lambda }_{1}}}\left[ \rho +\frac{\zeta ({{P}_{2}}\left( \sqrt{m} \right)+\bar{v}{{M}_{3}})}{2} \right] \right\}.\] |
利用反证法,假设当d2≥d2*时,系统(1)不存在非常数正平衡解.
由定理4可知,在d2≥d2*的条件下,存在正常数${{{\tilde{d}}}_{1}}$,使得当d1≥${{{\tilde{d}}}_{1}}$时,系统(1)无非常数正平衡解.并取d*1适当的大,使得d*1≥${{{\tilde{d}}}_{1}}$,0<$\frac{{{f}_{11}}}{{{d}_{1}}*}$<λ1.对于如此确定的(d*1,d2*),系统(1)无非常数正平衡解.
∀s∈[0,1],定义
| \[\begin{align} & D\left( s \right)=\left( \begin{matrix} s{{d}_{1}}+\left( 1-s \right){{d}^{*}}_{1} & 0 \\ 0 & s{{d}_{2}}+\left( 1-s \right){{d}_{2}}^{*} \\ \end{matrix} \right)= \\ & \left( \begin{matrix} {{d}_{1}}\left( s \right) & 0 \\ 0 & {{d}_{2}}\left( s \right) \\ \end{matrix} \right). \\ \end{align}\] |
其中由于d1≥${{{\hat{d}}}_{1}}$,d2≥d2*,d*1≥${{{\hat{d}}}_{1}}$,所以d1(s)>${{{\hat{d}}}_{1}}$,d2(s)≥d2*.
下面考虑如下问题
| \[\left\{ \begin{align} & -\Delta U={{D}^{-1}}\left( s \right)F\left( U \right),x\in \Omega , \\ & {{\partial }_{v}}U=0,x\in \partial \Omega . \\ \end{align} \right.\] | (9) |
则U是方程(9)的非常数正解,当且仅当U是
| \[W\left( U,s \right)=U-{{\left( I-\Delta \right)}^{-1}}({{D}^{-1}}\left( s \right)F\left( U \right)+U\] | (10) |
的解.其中
| \[\begin{align} & W\left( U,1 \right)=G({{d}_{1}},{{d}_{2}};U),W\left( U,0 \right)=G({{d}^{*}}_{1},{{d}_{2}}^{*};U), \\ & {{D}_{U}}G({{d}_{1}},{{d}_{2}};{{U}_{r}})=I-{{\left( I-\Delta \right)}^{-1}}(D{{\left( 1 \right)}^{-1}}{{J}_{r}}\left( U \right)+I),r=1,2, \\ & {{D}_{U}}G({{d}^{*}}_{1},{{d}_{2}}^{*};{{U}_{r}})=I-{{\left( I-\Delta \right)}^{-1}}(D{{\left( 0 \right)}^{-1}}{{J}_{r}}\left( U \right)+I),r=1,2. \\ \end{align}\] |
由先验估计可知,存在正常数K1,K2,使得∀s∈[0,1],方程(9)的正解满足K1<u(x),v(x)<K2,x∈${\bar{\Omega }}$.令
| \[\Sigma = \left\{ {U \in {{\left[ {C\left( {\bar \Omega } \right)} \right]}^2}|{K_1} < u(x),v(x) < {K_2},x \in \bar \Omega } \right\}.\] |
因此,∀s∈[0,1],W(U,s)=0在U∈∂Σ上无解.根据度的同伦不变性[12]可得
| \[\deg \left( W\left( U,1 \right),\Sigma ,0 \right)=\deg \left( W\left( U,0 \right),\Sigma ,0 \right).\] | (11) |
然而,由反证假设可知,当d2≥d2*时,W(U,1)=0在区域Σ上只有正常数解U1,U2,且
| \[\text{index}W({{U}_{1}},1)=\text{index}G({{d}_{1}},{{d}_{2}};{{U}_{1}})={{\left( -1 \right)}^{dimE\left( {{\lambda }_{0}} \right)+{{\sigma }_{q}}}}=1,\] | (12) |
| \[\text{index}W({{U}_{2}},1)=\text{index}G({{d}_{1}},{{d}_{2}};{{U}_{2}})={{\left( -1 \right)}^{0}}=1.\] | (13) |
结合定理4和上述d*1,d2*的取法可知,W(U,0)=0在区域Σ上只有正常数解U1,U2,且0<$\frac{{{f}_{11}}}{{{d}^{*}}_{1}}$<λ1,从而A1(d1*,d2*)∩Sp={λ0},因此
| \[\text{index}W({{U}_{1}},0)=\text{index}G({{d}^{*}}_{1},{{d}_{2}}^{*};{{U}_{1}})={{\left( -1 \right)}^{dimE({{\lambda }_{0}})}}=-1\] | (14) |
| \[\text{index}W({{U}_{2}},0)=\text{index}G({{d}^{*}}_{1},{{d}_{2}}^{*};{{U}_{2}})={{\left( -1 \right)}^{0}}=1.\] | (15) |
结合式(12)~(15)可得
| \[\begin{align} & \deg \left( W\left( U,1 \right),\Sigma ,0 \right)=\text{index}W({{U}_{1}},1)+\text{index}W({{U}_{2}},1)=2, \\ & \deg \left( W\left( U,0 \right),\Sigma ,0 \right)=\text{index}W({{U}_{1}},0)+\text{index}W({{U}_{2}},0)=0 \\ \end{align}\] |
这与式(11)矛盾,故假设不成立,定理5得证.
记${\sigma _q} = \mathop \sum \limits_{i = 1}^q dimE({\lambda _i}),{\sigma _p} = \mathop \sum \limits_{i = 0}^q dimE({\lambda _i})$,类似地有如下定理.
定理 6 设$1 - \frac{h}{c} < \frac{\alpha }{\beta } < 1 + c - 2\sqrt h < 1 - c$成立,且f21>0.如果存在d1满足∀q≥1,p≥0,都有$\frac{{{f_{11}}}}{{{d_1}}} \in ({\lambda _q},{\lambda _q} + 1),\frac{{{f_{21}}}}{{{d_1}}} \in ({\lambda _p},{\lambda _{p + 1}}),$,σq+σp为奇数,则存在d2*>0,使得当d2≥d2*时,系统(1)至少存在一个非常数正平衡解.
证明 因为f21>0,g22<0,f21g22-f22g21>0,可以得到λ2+>0,λ2->0,且$\mathop {\lim }\limits_{{d_2} \to \infty } \lambda _2^ + = \frac{1}{2}\frac{{{f_{11}}}}{{{d_1}}} > 0,\mathop {\lim }\limits_{{d_2} \to \infty } \lambda _2^ - = 0$,从而存在${{{\hat d}_2}}$,使得0<λ2-<λ1,λp<λ2+<λp+1,从而存在足够大的1,使得当d1≥1时,0<λ2+<λ1.则类似定理5的证明,可证得本定理.
| [1] | 陈兰荪.数学生态学建模与研究方法[M].北京:科学出版社,1988:1-126. CHEN Lansun.Mathematical ecology modeling and research method[M].Beijing:Science Press,1988:1-126. |
| Click to display the text | |
| [2] | ANDREWS J F.A mathematical model for the continuous culture of microorganisms utilizing inhibitory substrates[J].Biotechnol Bioeng,1968,10(6):707-723. |
| Click to display the text | |
| [3] | CLARK C W.Mathematical models in the economics of renewable resources[J].SIAM Rev,1979,21(1):81-99. |
| Click to display the text | |
| [4] | KRISHNA S V,SRINIVASU P D N,KAYMACKALAN B.Conservation of an ecosystem through optimal taxation[J].Bull Math Biol,1998,60(3):569-584. |
| Click to display the text | |
| [5] | DAS T,MUKHERJEE R N,CHAUDHURI K S.Bioeconomic harvesting of a prey-predator fishery[J].Biol Dyn,2009,3(5):447-462. |
| Click to display the text | |
| [6] | ZHANG J H,YANG C H,ZHANG X G,et al.Bifurcation analysis in a predator-prey system with Holling-Ⅳ functional response and prey constant harvesting[J].Int J Inf Syst Sci,2012,3(8):382-395. |
| Click to display the text | |
| [7] | GUPTA R P,CHANDRA P.Bifurcation analysis of modified Leslie-Gower predator-prey model with Michaelis-Menten type prey harvesting[J].J Math Anal Appl,2013,398(1):278-295. |
| Click to display the text | |
| [8] | LI Yan,WANG Mingxin.Dynamics of a diffusive predator-prey model with modified Leslie-Gower term and Michaelis-Menten type prey harvesting[J].Acta Appl Math,2015,140(1):147-172. |
| Click to display the text | |
| [9] | LOU Yuan,NI Weiming.Diffusion vs cross-diffusion:An elliptic approach[J].J Differential Equations,1999,154(1):157-190. |
| Click to display the text | |
| [10] | LIN Chuangsheng,NI Weiming,TAKAGI I.Large amplitude stationary solutions to a chemotaxis system[J].J Differential Equations,1988,72(1):1-27. |
| Click to display the text | |
| [11] | GILBARG D,TRUDINGER N S.Elliptic partial differential equations of second order[M].New York:Springer Verlag,1983:20-71. |
| Click to display the text | |
| [12] | YE Qixiao,LI Zhengyuan.Introduction to reaction diffusion equations[M].Beijing:Science Press,1990:1-48. |
| Click to display the text | |
| [13] | 徐娟,李艳玲.一类捕食-食饵模型正平衡解的存在性[J].纺织高校基础科学学报,2012,25(2):149-153. XU Juan,LI Yanling.The existence of positive steady-state solutions for a predator-prey model[J].Basic Sciences Journal of Textile Universities,2012,25(2):149-153. |
| Cited By in Cnki (4) | |
| [14] | 张岳,李艳玲.带有交叉扩散项的 Holling-Ⅱ捕食-食饵模型的共存[J].纺织高校基础科学学报,2010,23(4):439-444.[JP] ZHANG Yue,LI Yanling.Stationary patterns for a prey-predator model with Holling type II functional response and density-dependent diffusion term[J].Basic Sciences Journal of Textile Universities,2010,23(4):439-444. |
| Cited By in Cnki (13) | |
| [15] | PANG P Y H,WANG Mingxin.Non-constant positive steady states of a predator-prey system with non-monotonic functional response and diffusion[J].Proc London Math Soc,2004,1(1):135-157. |
| Click to display the text |



